Laplace Transform Table | Phase delay 7 references 8 the following table provides laplace transforms for many common functions of a single variable. Mathematicians have developed tables of commonly used laplace transforms. Laplace transform function, table, propertiesand examples. This section is the table of laplace transforms that we'll be using in the material. While tables of laplace transforms are widely available, it is important to understand the properties of the laplace transform so that you can construct your own table.
By default it will return conditions of convergence as well (recall this is an improper integral, with an infinite bound. Before actually talking about laplace transforms, there are a couple of questions to answer. The following is a list of laplace transforms for many common functions of a single variable. The given ode is transformed into. Y(t) , definition of laplace transform.

Its laplace transform (function) is denoted by the corresponding capitol letter f. Why do we need laplace transforms? Laplace transform example find l(e5t (t + 2)2). Laplace transforms of elementary functions. In this lesson, we will generate a table of laplace transforms and their inverses that will be used to solve subsequent problems. Mathematicians have developed tables of commonly used laplace transforms. In this topic, you study the table of laplace transforms. Laplace transform with examples and solutions find area under curve table of integrals properties of integrals. The given ode is transformed into. The above worksheet is a walk through to understand the concept of laplace transform, properties and table. Table of laplace transforms revision j. Phase delay 7 references 8 the following table provides laplace transforms for many common functions of a single variable. Revised with corrections july 1, 2016.
Revised with corrections july 1, 2016. Before actually talking about laplace transforms, there are a couple of questions to answer. The given ode is transformed into. Moreover, the laplace transform converts one signal into another conferring to. Y(t) , definition of laplace transform.
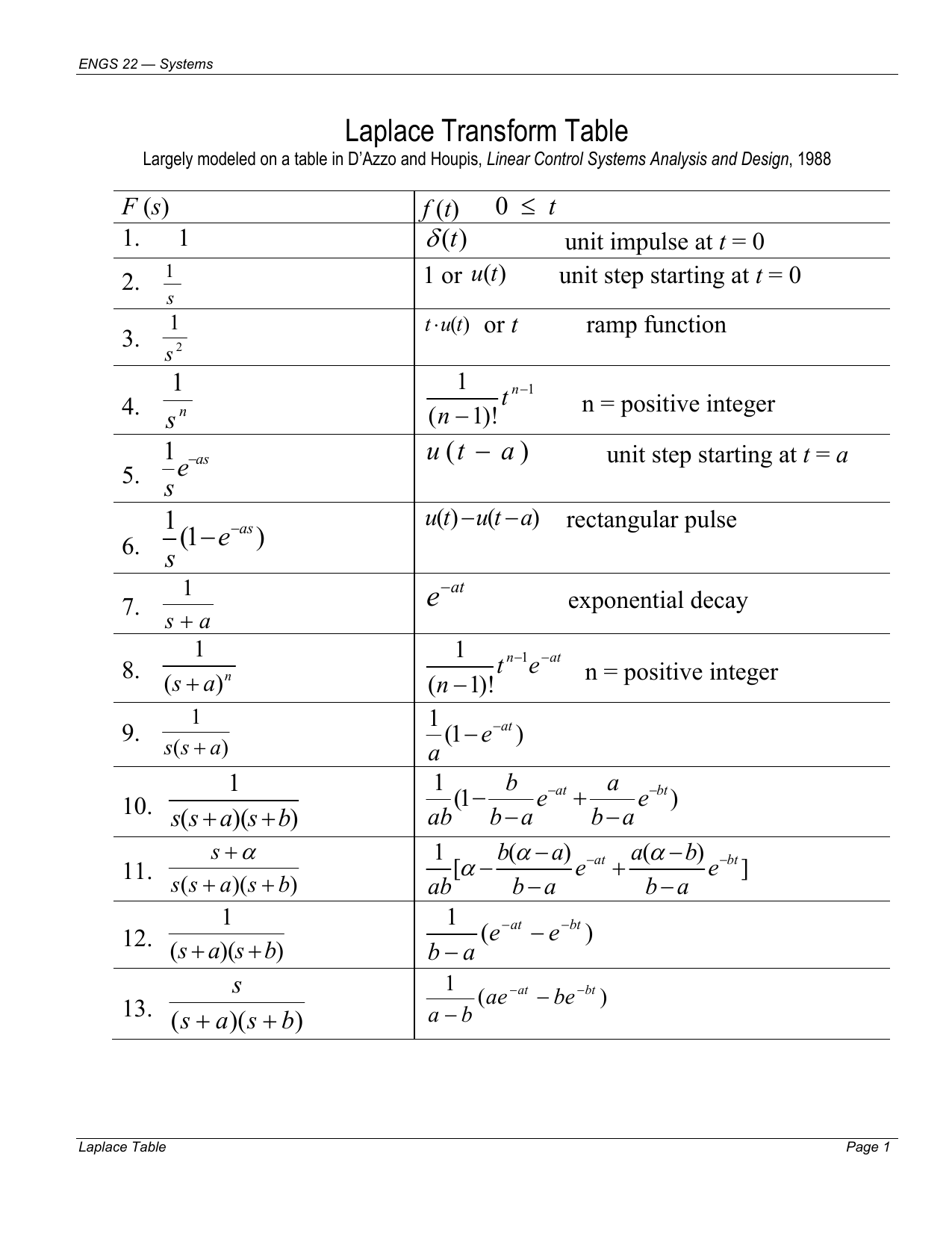
The given ode is transformed into. Mathematicians have developed tables of commonly used laplace transforms. Revised with corrections july 1, 2016. Each expression in the right hand column (the laplace. To basically simplify the method of solving a lot of problems. Y(t) , definition of laplace transform. While tables of laplace transforms are widely available, it is important to understand the properties of the laplace transform so that you can construct your own table. Laplace transform example find l(e5t (t + 2)2). Before actually talking about laplace transforms, there are a couple of questions to answer. The process of solving an ode using the laplace transform method consists of three steps, shown schematically in fig. Its laplace transform (function) is denoted by the corresponding capitol letter f. Laplace transform of $f(t)$ is. The name 'laplace transform' was kept in honor of the great mathematician from france, pierre simon de laplace.
The given ode is transformed into. Laplace transform example find l(e5t (t + 2)2). The actual laplace transform is often done using the laplace transform table. Using above property, the laplace transform of basic functions are. Moreover, the laplace transform converts one signal into another conferring to.
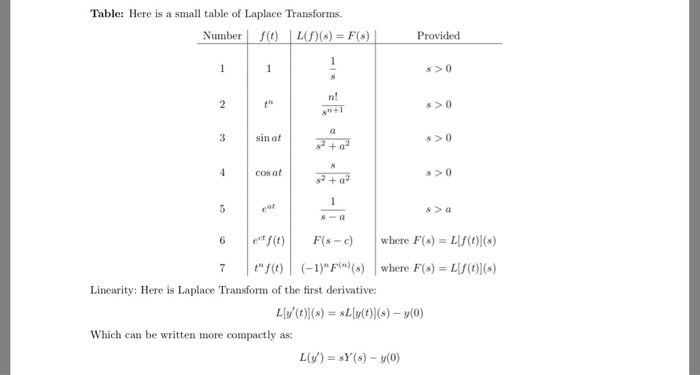
(often time) to a function of a complex variable. It's time to stop guessing solutions and nd a systematic way of nding solutions table 1 below provides the laplace transform for many common functions. The actual laplace transform is often done using the laplace transform table. We give as wide a variety of laplace transforms as possible including some that aren't often given in tables of laplace. Laplace transforms of elementary functions. Table of laplace transforms revision j. The following is a list of laplace transforms for many common functions of a single variable. Why do we need laplace transforms? Table of elementary laplace transforms. Using above property, the laplace transform of basic functions are. In this lesson, we will generate a table of laplace transforms and their inverses that will be used to solve subsequent problems. In this topic, you study the table of laplace transforms. The above worksheet is a walk through to understand the concept of laplace transform, properties and table.
Laplace Transform Table: Sympy provides a function called laplace_transform which does this more efficiently.
Referanse: Laplace Transform Table
0 comments:
Post a Comment